Geometry / Planetary Gears¶

Description: Review the geometric design of planetary geartrain layouts.

Table of Contents¶

Introduction
Standard Planetary
Compound Planetary
References

Introduction¶

The geometry of planetary geartrain layouts is reviewed here. Planetary geartrains can be configured in several ways, with the most common architectures reviewed here. Attractive characteristics of planetary geartrains include:

Mechanical power is transferred between concentric bodies (ring, sun, carrier)
Power density is high, providing great mechanical power in a small package
Multiple gear meshes act on central bodies, with self-centering tendencies
Planet gears may precess with the carrier reference frame
Cylindrical involute gears are used to transmit motion
Multiple power inputs or outputs can be defined

The visualization of a planetary geartrain clearly demonstrates its unique geometric characteristics.

Spur planetary geartrain

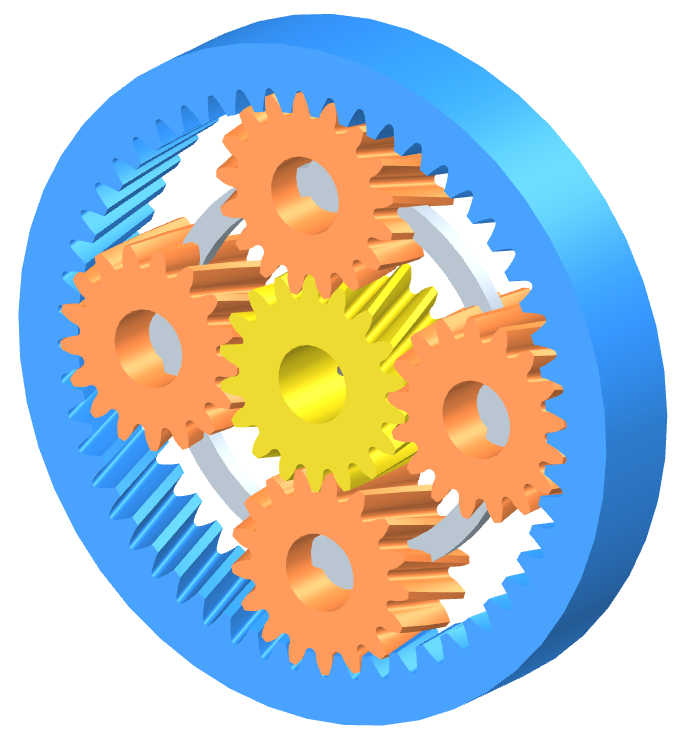
Planetary geartrain modeled in Gears App

Most commonly, planetary geartrains have a sun gear (yellow), ring gear (blue), and multiple planet gears (orange) on a carrier (gray). Spur or helical gears are used for a planetary gearset, with each type having advantages. This notebook reviews the unique geometric attributes of planetary gears and their significance to planetary gear mesh operation.

The assembly of a planetary geartrain is dependent on various geometric attributes, such as tooth counts.

The various conditions that must be satisfied for a planetary geartrain to successfully assemble are reviewed in this notebook. To understand the geometry of the spur or helical gears that makeup a planetary geartrain, see the respective notebooks on gear geometry.

Standard Planetary¶

This section reviews the design layout of standard planetary geartrains, i.e. planetaries with a single sun, ring, and set of planets like shown in the image below. Working examples are provided for spur and helical planetary geartrains.

Helical planetary geartrain

Standard planetary modeled in Gears App

Nomenclature ¶

This table provides a set of parameters commonly used to define the geometry of planetary geartrains.

Symbol	Description
$i$	Transmission ratio
$u$	Base ratio
$\omega$	Angular velocity
$m_n$	Normal module
$z$	Number of teeth
$\beta$	Helix angle
$d_a$	Tip diameter
$p_t$	Transverse pitch
$d_w$	Pitch diameter
$a$	Center distance
$l$	Planet distance
$N$	Number of planets

For a planetary geartrain, the subscripts _$1$, _$2$, _$3$, and _$V$ are used for the sun, planet, ring, and carrier, respectively.

Ratios ¶

When designing a planetary geartrain, a transmission ratio is typically used to define a target or goal. The design space is largely determined from the target ratio, so an allowable deviation is often used depending on the application requirements. The general equation for transmission ratio is expessed as:

$$i = \frac{\omega_\text{in}}{\omega_\text{out}}$$

A base ratio is defined as the transmission ratio between the sun and ring gear, with a fixed carrier. This is effectively an idler geartrain, with the planets behaving as idler gears. The ratio is calculated as:

$$u = \frac{z_3}{z_1}$$

where sign convention for number of ring gear teeth, $z_3$, is negative. The transmission ratio between the sun gear and carrier, with the ring gear constrained to ground, is expressed as:

$$i_{1V} = 1 - u$$

Additionally, the transmission ratio between the carrier and ring gear, with the sun gear constrained to ground, is expressed as:

$$i_{3V} = 1 - \frac{1}{u}$$

Other transmission ratios of interest may be solved for by rearranging the equations above.

Planetary geartrains are capable of having non-zero angular velocity for all components.

To learn more about the fundamentals of rotational kinematics and how gears transfer motion, refer to our kinematics notebook. The current notebook is focused on planetary design layouts.

Constraints ¶

To design a valid planetary geartrain, one that can be assembled and operate in real-world applications, multiple constraints must be adhered to. All the constraints relate to geometrical relationships of planetary geartrains.

Geometric layouts are used to study the feasible design space for planetary geartrains.

As will be shown, only certain combinations of tooth counts, planet count, and planet spacing are possible.

Center Distance¶

The geometric condition that requires the center distance for the sun-planet and planet-ring gear meshes to be the same is obvious when illustrated. The equation to express this relationship can be expanded as a function of the working pitch diameters:

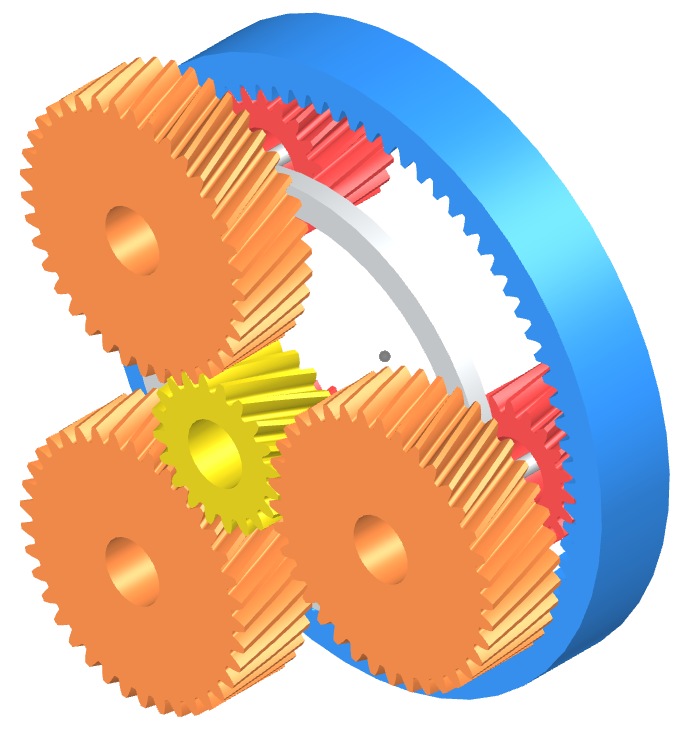
$$\lvert a_{12} \rvert = \lvert a_{23} \rvert$$$$\lvert d_{w1} + d_{w2} \rvert = \lvert d_{w2'} + d_{w3} \rvert$$

where $d_{w2'}$ specifically refers to the working pitch diameter of the planet-ring gear mesh, which may differ from the sun-planet mesh due to profile shifts, tooth counts, and center distance differing from nominal (a condition with zero profile shift or backlash). Below illustrates a layout diagram for a 4-planet geartrain:

Planetary layout diagram

Planetary layout diagram of 4-planet geartrain

Analogous to an idler gear, the planet gear has no effect on the planetary transmission ratios.

When considering the detailed tooth form of the meshing gears, this means multiple values of planet tooth count are possible for a given design. The design space is somewhat complicated by this, but it is more applicable to the detailed tooth design, so does not require immediate consideration.

Planet Interference¶

Upon viewing the enveloping geometry, notice the condition of planet-to-planet interference must be considered. Clearance must exist between the tip diameters of each planet. The equation to express this constraint is:

$$l > d_{a2}$$

where $l$ is the center distance between planets, expressed as:

$$l = 2a\sin\left(\frac{\pi}{N}\right)$$

In the conceptual design stage, tip diameters are usually unknown, so the pitch diameter may be used as an initial approximation.

Meshing Teeth¶

Despite a given enveloping diagram appearing as a valid design, remember that an integer number of teeth is required for all the gears. Adding to this requirement, the teeth of each planet must mesh with both the sun and ring. Determining valid conditions for teeth to mesh depends on the tooth counts, number of planets, and planet spacing.

To understand this requirement, let's imagine assembling a planetary geartrain. First, position the sun at the origin with any angle of rotation. Next, add the first planet to a desired carrier position, rotating its teeth to mesh with the sun. Then, position the ring at the origin, rotating its teeth to mesh with the planet. Now, imagine adding the second planet somewhere on the carrier, not being allowed to rotate the sun or ring. Only certain carrier positions will allow the second planet to mesh with both the sun and ring.

Equal Spacing¶

For equally-spaced planets, the following equation is used to identify valid meshing conditions for a given number of planets and tooth counts:

$$k = \frac{z_1 - z_3}{N}$$

where $k$ must be an integer for the gears to mesh.

Unequal Spacing¶

It is also possible for the gears to mesh with unequal planet spacing. In such cases, the smallest angular unit pertaining to the allowable planet positions is used to find the planet positions nearest equal spacing. This unit angle of planet position, or so-called tick angle, may be calculated as:

$$\hat{\theta} = \frac{2 \pi}{z_1 - z_3}$$

Typically, unuequal spacing is only used in 4-planet geartrains where the planet gears can be positioned with diametrically-opposed spacing. Sometimes this is called an X configuration. A diametrically-opposed design is allowed if the remainder of $k$ is 0.5. Designs capable of being equally-spaced can also be modified to be diametrically-opposed.

Mesh Phasing¶

Once the geometric conditions are met to ensure a valid planetary geartrain can be assembled, it is also of interest to consider the phasing of the planetary gear meshes. Phasing refers to the cyclic behavior of multiple gear meshes with the same frequency.

Three types of planetary phasing exist for nominal conditions.

In-phase
Sequential-phase
Counter-phase

Before explaining each phasing type, it must understood that phasing specifically refers to the phase angle for the harmonics of gear mesh excitation caused by imperfect conjugate action, commonly referred to as transmission error. These excitations are caused by numerous things, including manufacturing and assembly errors, but also due to the deflection of gear teeth under operating loads.

Gear mesh excitations can be viewed as a series of sinusoidal signals, called harmonics, each with an amplitude and phase angle.

Fourier transform is used to analyze a time signal as a series of sinusoids, useful for harmonic analysis.

Phase Angles¶

To understand the different types of planetary phasing, we must first consider the phasing of each gear mesh in the planetary geartrain. Let's individually examine the gear meshes for the ring and sun.

Only the relative phase angles are of significance for planetary phasing analysis.

Planet-Ring¶

To determine the phase angle of a planet-ring mesh, we must define a relationship between the position of ring gear teeth and the position of the planet gear, e.g. equally-spaced or diametrically-opposed. Successive ring gear teeth are separated by the ring transverse pitch angle, defined as:

$$\psi_{t3} = \frac{2 \pi}{z_3}$$

By writing a fraction that divides the $i^\text{th}$ planet angle by the ring transverse pitch angle,

$$q_{3i} = \frac{\theta_i}{\psi_{t3}}$$

the mesh phase angle is determined by the remainder of $q_{3i}$, the fraction of mesh cycle, according to:

$$\phi_{3i} = 2 \pi * (q_{3i} \bmod 1)$$

Sun-Planet¶

Following a similar logic as above, we can compute the sun-planet gear mesh phase angles as with equations:

$$\psi_{t1} = \frac{2 \pi}{z_1}$$$$q_{1i} = \frac{\theta_i}{\psi_{t1}}$$$$\phi_{1i} = 2 \pi * (q_{1i} \bmod 1)$$

Phasing Types¶

The phasing classification for a planetary geartrain is based on the first harmonic of its gear mesh excitations. Higher harmonics may have different phasing. The following equation can be used to determine phasing with equally-spaced planets:

$$k_\phi = |z_3| \bmod N$$

In particular, the phasing is of interest to understand which gear mesh excitations reinforce or cancel in the system. Excitation reinforcements and cancellations are studied for the following summed components:

Torques and axial forces, i.e. thrusts (helical only)
Radial forces

In-Phase¶

In-phase refers to having the same phase angle for each gear mesh. In-phase planetaries have reinforced torques and thrusts but cancelled radial forces. The condition for in-phase is:

$$k_\phi = 0$$

Sequential-Phase¶

Sequential-phase refers to having equally separated phase angles for subsequent gear meshes. Sequential-phase planetaries have cancelled torques and thrusts but reinforced radial forces. The condition for sequential-phase:

$$k_\phi = 1, N-1$$

Counter-Phase¶

Counter-phase refers to having opposite phase angles for opposing planets. Counter-phase planetaries have all summed components of excitation cancelled. The condition for counter-phase is:

$$k_\phi = 2, 3, \dots, N-2$$

Mixed-Phase¶

Mixed-phase refers to having a combination of phasing types. This can only occur with unequally-spaced planets, as found in diametrically-opposed designs. In such cases, the gear mesh phase angles must be calculated and used in the sum of harmonics to determine which reinforcements and cancellations occur.

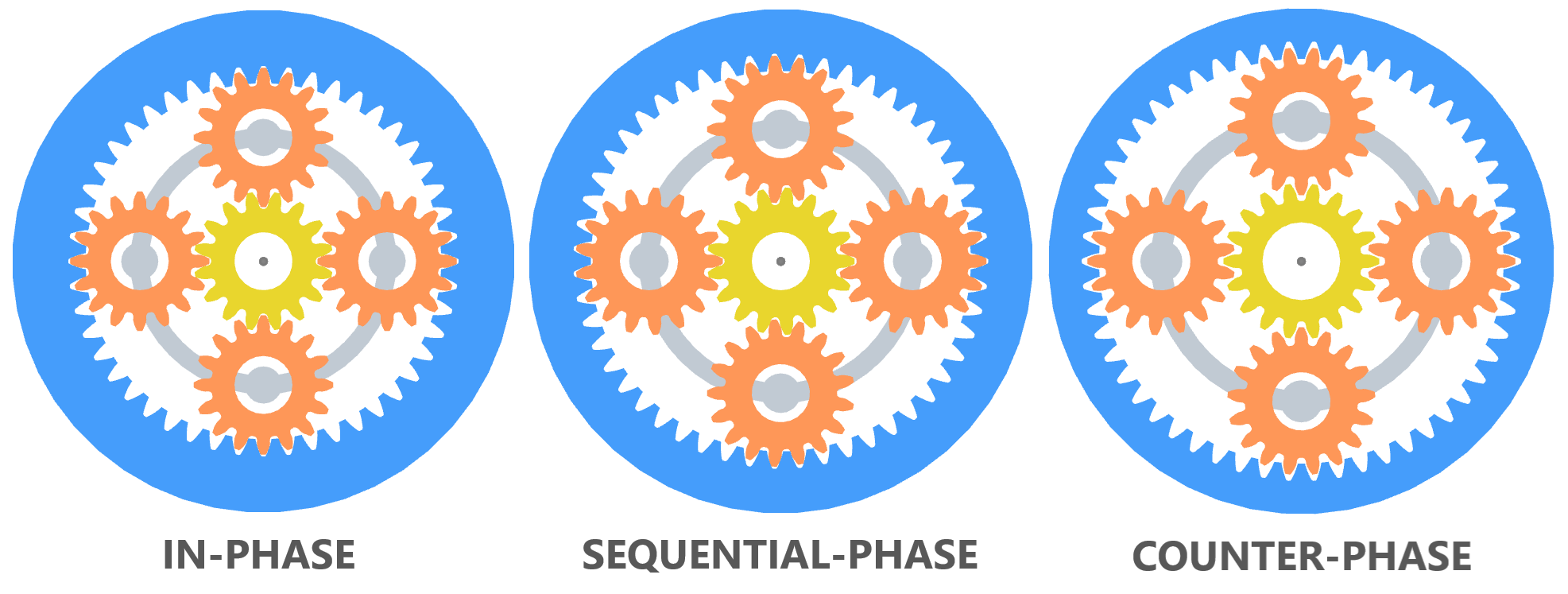
Compare planetary phasing types

Planetary geartrain phasing types modeled in Gears App

Perfect planetary phasing conditions are not observed in real-world conditions.

Errors caused by manufacturing, assembly, and operation can cause gear mesh phasing deviations and low frequency modulations that will lead to more vibration frequencies, increased vibrations, and undesireable noise characteristics. Planetary phasing should be considered during the design-phase.

Helical Gears¶

To extend the planetary design rules to helical gearing, we need to consider the helix angle and the transverse geometric properties, such as module and pitch diameters. The transverse module of a helical gear is most easily understood as the millimeters of theoretical pitch diameter per tooth:

$$m_t = \frac{d}{z}$$

which can be expressed as a function of the normal module and helix angle according to:

$$m_t = \frac{m_n}{\cos\beta}$$

It can be deduced from the equations above that for a given normal module and number of teeth, increasing the helix angle causes an increase in theoretical pitch diameter. This is important to consider for planetary layout design.

Phasing¶

For the fundamentals of planetary phasing, the introduction of a helix angle does not change a lot. The concept is still the same, i.e. each planetary gear mesh produces a dynamic signal at the mesh frequency and these signals may cancel or reinforce in various ways. Since a helix angle introduces axial forces into the gear mesh, it may also be considered in the cancellations and reinforcements.

The sum of axial forces cancel / reinforce the same as torques.

To learn more about the detailed attributes of helical gears, see our notebook on helical gears and gear meshes.

Example | Standard Planetary

GIVEN

Target transmission ratio, with +/- range.
Target ring gear diameter.
Target number of planets.
Range of helix angles.

FIND

Standard planetary designs that satisfy the input constraints.

SOLUTION

See below.

Compound Planetary¶

This section reviews the design layout of compound planetary geartrains with a sun, ring, and planet shafts each with two planet gears. Reference the image below. Working examples are provided for spur and helical planetary geartrains.

Compound planetary geartrain

Compound planetary modeled in Gears App